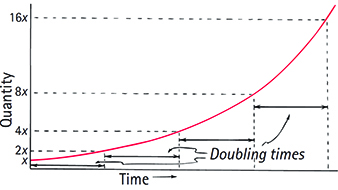
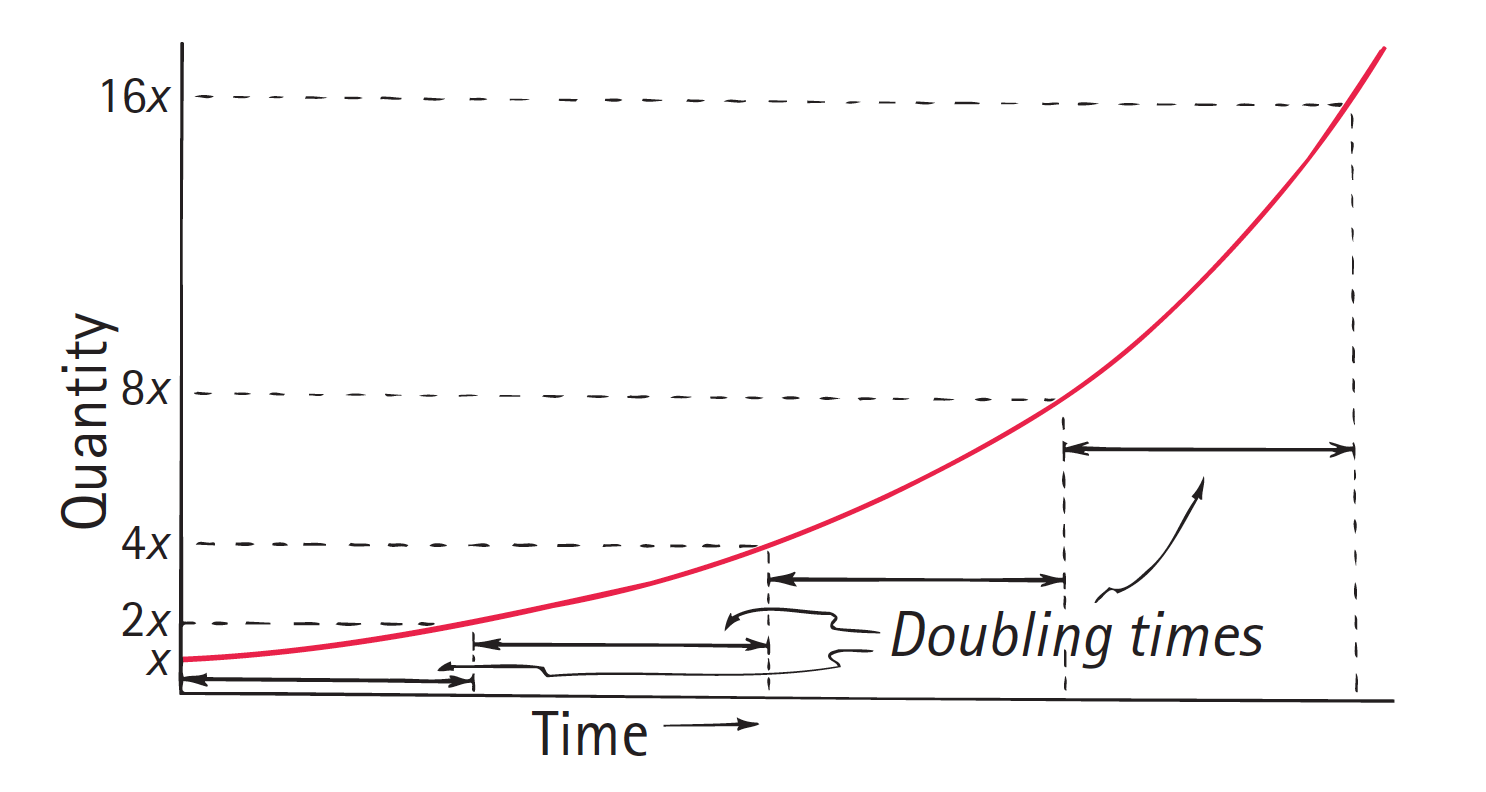
A Function Has a Constant Doubling Time

Price holding all else constant. To model the population at any arbitrary year we rewrite the exponential function in terms of the doubling time.

Exponential Growth And Doubling Time Nsta

A₀ is the initial amount h is the half life time or the halving time.

. Every twelve years the population doubles and the exponent becomes an integer based on nt12. Ft300cdot 2t12 or more generally ft300cdot 2tT where T is the doubling time. We have a function of the form ft A3tT and you can see that.

As y doubles proportionately to x. Radioactive decay is a good example where the half life is constant over the entire decay time. Exponential growth O c.

Holding all else constant. It is proved in calculus that this law requires that the quantity is given by the exponential function if we use the correct time scale. If you dont know your growth rate you can derive it by subtracting your past quantity from your current quantity and dividing the result by.

Depends it can be true or false Apex. Noting that for i 0 1 the corresponding term inside the sum would be zero we can start the sum at i 0 without affecting anything. Making this substitution we have.

An exponential growth function represents a quantity that has a constant doubling time. The rule of 72 is found by dividing 72 by the rate of interest expressed as a whole number. To calculate the doubling time using the rule of 70 we have dt 70 14 5.

What type of function does the represent. View results An exponential function is written as Fx equals a bx where the coefficient a is a constant the base b is but not equal to 1 and the exponent x is any number. The graph illustrates how exponential growth.

A function has a constant doubling time. We can additionally expand and. F0 A fT A3 f2T A32 A33 and so on.

Ft300cdot 2t12 or more generally ft300cdot 2tT where T is the doubling time. If cd a 1 de 2a 1 st 2b 3 and tu. EqDoubling time ln2nln1rn eq.

When x3 y doubles or y 8. To model the population at any arbitrary year we rewrite the exponential function in terms of the doubling time. This is just i i 1 2.

Whereas a demand function is Quantity vs. In non-exponential decay half life is not constant. Other questions on the subject.

For an exponentially growing quantity the value Q of the function is 2Upper Q 0Q0 after one doubling time 4Upper Q 0Q0 after two doubling times 8Upper Q 0Q0 after three doubling times and so on. What type of function does this represent. Doubling time can found using the doubling time formula.

When x2 y doubles or y 4. Doubling time is the amount of time it takes for a given quantity to double in size or value at a constant growth rate. C n i 2 n 1 i 2 i 1 2.

The doubling time formula is. For example if the population of a growing city takes 10 years to double from 100000 to 200000 inhabitants and its growth remains exponential then in the next 10 years the population will double to 400000 and. That is y output doubles with x input y tex 2 x jd3sp4o0y and 12 more users found this answer helpful.

An exponential decay function represents a quantity that has a constant doubling time. To do this we divide 70 by the growth rate r. An exponential decay function represents a quantity that has a constant doubling time.

Exponential decay O B. The importance of the exponential curve of Figure 1 is that the time required for the growing quantity to double in size a 100 increase is a constant. As stated this is only an estimation as a 6 rate would take 1190 years using the actual doubling time formula.

This is an exponential function. A quantity is said to be subject to exponential decay if it decreases at a rate proportional to its value. The only non-exponential function that has this property would be a function that has the constant value of zero.

This explains the name. What type of function does this represent. For example a rate of 6 would be estimated by dividing 72 by 6 which would result in 12 years.

Every twelve years the population doubles and the exponent becomes an integer based on nt12. To calculate doubling time first multiply your growth rate by 100 to convert it to a percentage. What the difference between an exponential equation and a power equation.

Decreasing linear O D. The time required for the decaying quantity to fall to one half of its initial value. Mathematics 21062019 1400 miriamnelson7545 cde maps to stu with the transformations x y arrowright x 2 y 2 arrowright 3x 3y arrowright x y.

When x1 y doubles or y 2. A function has a constant doubling time. A function has a constant doubling time.

Then if A is the initual cuantity. Y ax where a is. An exponential decay function represents a quantity that has a constant doubling time.

We can find the doubling time for a population undergoing exponential growth by using the Rule of 70. This means that the population doubles every. So the doubling time of the rabbit population is 5 years.

Depends it can be true or false Apex. If you can imagine a graph with the y-axis being Price and the x-axis being Quantity and you were to. A constant tripling time means that there is a time T a quantity triples when t T then triples again when t 2T and again when t 3T.

3 Show answers Another question on. Is the initial value of the function. Depends it can be true or false Apex.

T is the time At the amount that remains at time t The previous function represents an. Fit a curve between these points. The function which has a constant halving time is in the following form Where.

Exponential Growth And Doubling Time Nsta

Doubling Time Calculator Formula Equation

Solved True False Please Select True Or False And Click Chegg Com

Find The Doubling Time Of Exponential Growth Youtube